The techniques of optimizations are significant tools needed for measuring the efficiency of business firms. The economic agents have different motives and they all want to optimize such motives at the cost of their resources. Here we will talk about the basic concept and rules of the derivative as a technique for solving or analyzing the optimization problems of economic agents.

Learning Objective To discuss the concept and rules of derivative

The Concept of Derivative

The concept of the derivative is mainly used in problems that are concerned with the changes occurring in the variables. Functions like cost, demand, supply, profit, revenue, growth, etc. usually change under certain conditions and we have to analyze the changes occurring in these functions in economics and business management. The derivative ΔY/ΔX measures the rate of change of the variable y concerning the variable x on which it depends. So the changes occurring in the variables mentioned above are also described in terms of their derivatives. Therefore, the concept rules of the derivative are much useful in economics and business decision-making.

Derivative also explains the slope of the continuous and smooth non-linear curve. If the given function is Y=f (X), then ΔY/ΔX measures the slope of the function at a particular point and it is known as derivative dY/dX of the function concerning X. The derivative dY/dX (more especially the first-order derivative) of a function defines the limit of ratio ΔY/ΔX as ΔX approaches to zero.

The slope of a function (linear or non-linear) is determined by derivative dY/dX and brings a change in the dependent variable due to a small change in the independent variable. This concept has a very wider application in economics and managerial decision-making. The issues related to profit maximization; sales revenue maximization, cost minimization, output maximization, etc. are solved with the help of the concept of the derivative.

Rules of Differentiation

Differentiation is the procedure of deducing the derivative of a given function. The derivative of any function signifies the change in the dependent variable as a result of a small change in the independent variable. It is expressed as dY / dX for a function Y = f (X). There are several rules of differentiation and several sorts of functions. Major rules are discussed below;

Constant Rule

A constant function is given as Y=f(X) = j; Where ‘j’ is a constant. It implies that the value of Y will not fluctuate as there is a change in the value of X. It means Y is not depending on X. So, the derivative of a constant function is always zero. It is given as; dY/dX = 0

Power Rule

The standard power function is; Y= aX^b; Where ‘a’ and ‘b’ both are constant. In our function, ‘a’ is the coefficient of independent variable X, and this variable is raised to the exponent b. The derivative of the given function according to the power rule is;

dY/dX= d(aX^b)/dX= b.a.X^b-1

Derivative in Case of Sum or Difference of Two Functions

If the functions are given in the form of Y=f(X) ±g(X), where f(X) and g(X) are two unspecified functions and Y is the summation of these two functions. Now we can obtain the derivative by using the following technique;

dY/dX= d f (X)/dX ± d g(X)/dX

For instance,

A). Let Y= 3X⁵+5X³

Now, dY/dX= d(3X⁵+5X³)/dX= 5*3X^5-1 + 3*5X^3-1 =15X⁴+ 15X²=15(X²+X⁴)

B). Let Y=5X³-2X⁵

Now, dY/dX= d(5X³-2X⁵)/dX= 3*5X^3-1 -5*2X^5-1 =15X²– 10X⁴

Product Rule

Let us assume that Y is the product of the two separate functions f (X) and g(X) as Y=f (X). g (X).

Now the derivative of the product of the given two functions is just equivalent to the sum of an initial function multiply the derivative of the second function and the second function multiply the derivative of an initial function. Symbolically;

dY/dX= f(X). {dg(X)/dx}+ g(X).{d f(X)/dX}

For instance,

Let, Y=3X² (2X+3)

Here, let f (X) = 3X² and g(X) = (2X+3). Then the derivative under the product rule can be defined as;

dY/dX= 3X².{d(2X+3)/dx}+ (2X+3).{d(3X²)/dX}

Or, dY/dX= 3X².2+ (2X+3)2*3X^2-1

Or, dY/dX= 6X²+ (2X+3)6X

Or, dY/dX= 6X²+ 12X²+18X

Or, dY/dX= 18X²+ 18X

Or, dY/dX= 18(X²+X)

Quotient Rule

If Y is equal to the proportion or quotient of the two functions f(X) and g(X) then the rule of differentiation is called the quotient rule. The derivative under this rule is identical to the denominator times the derivative of the numerator minus the numerator times the derivative of the denominator and the whole divided by the denominator squared. Symbolically,

dY/dX= {g(X)*df(X)/d(X)-f(x)*dg(X)/d(x)}/{g(X)}²

For instance,

Let, Y=5X+2/X-1

Now, let us assume f(X) = 5X+2 and g(X) = X-1 then the derivative of the given function can be simplified by using the quotient rule as;

dY/dX= d (5X+2/X-1)/dX

Or, dY/dX= {(X-1)*d (5X+2)/d(X) – (5X+2)*d(X-1)/d(x)}/(X-1)²

Or, dY/dX = {(X-1)*5 – (5X+2)*1}/(X-1)²

Or, dY/dX = {(X-1)*5 – (5X+2)*1}/(X-1)²

Or, dY/dX=5X-5-5X-2/(X-1)²

Or, dY/dX=-7/(X-1)²

Chain Rule

If the variable Y is the function of a variable U and U is related to another variable X, and if we want to get the derivative of Y concerning to X, then we have to use another rule called the chain rule.

Let us assume that the variable Y is the function of variable U (Y=f (U)) and the variable U is a function of another variable X (U=g(X). If it is the case and then we can obtain the derivative of Y relating to change in X as denoted by dY/dX, we have to find the derivative of the two functions, Y=f (U) and U=g(X) separately and then multiply them together. Therefore,

For instance,

Let, Y=U³+15 and U=3X²

As we know, dY/dX= (dY/dU)*(dU/dX), so

Or, dY/dX= {d (U³+15)/dU}*{d (3X²)/dX}

Or, dY/dX= 3U²*6X

Substituting U= 3X² in the above equation, we get

dY/dX =3(3X²)²*6X= 3.9X⁴.6X =27X⁴.6X=162X⁵

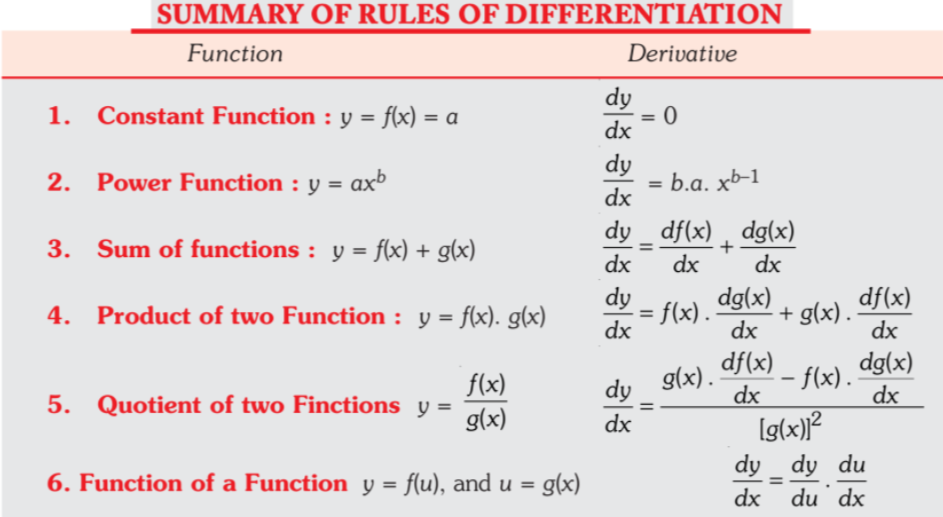
The following table summarizes the concept and rules of derivatives.

References and Suggested Readings

Ahuja, H.L.(2017). Advanced Economic Theory. Delhi: S Chand and Company Limited